Normal distribution

Probability

The probability that the tossed coin will fall with an eagle up 50%, that when the hexagon dice is thrown, 4 - 16.7% will fall, that tomorrow a meteorite will fall on someone - 0.00000000294%. These are simple examples, it is enough to divide the number the desired events for the total number of cases and we get the probability of the event, but when the results of the experiment can be not only heads or tails (which is equivalent to yes / no), but a large set of data. For example, the weight of a loaf of bread, if we take 1000 loaves of bread in the store and weigh each one, we will find out that in fact, the loaf does not weigh 400 grams, the results will vary in the range of 384-416 grams (the tolerance of the weight spread is provided by GOST). If you plot the "Number of loaves - Weight" graph, then the graph will have a bell-like shape, something similar to the following graph:

Probability density of the normal distribution

probability of normal distribution (graph)

The graph will get this form because most of the values are close to 400. This is an example of a normal distribution, a set of events have a law normal distribution, for example, weight or height for a certain age, or the average time of your trip to the store, and many other events also obey the law of normal distribution.

This is how marketers work: they conduct a survey of 1000 people and get an idea of the entire population

In the case of a table, you are dealing with discrete data, i.e. there is a certain probability for each weight, but in the case of a graph, the case changes a little, now we are not talking about 1000 loaves that we weighed, but about all the loaves in the world at once! What for? So as not to weigh all the loaves. Having the distribution law that we obtained by weighing 1000 loaves (we could weigh 100, 200, 500, as many as we like), we can assume, that no matter how many loaves we take, measuring them, we will get the same bell shape. Using statistical terms, all loaves of bread are a general population, 1000 measured loaves are a sample.

Now, let's take one loaf of bread, what is the probability that its weight will be between 390g and 400g?

Probability of an event between a and b:
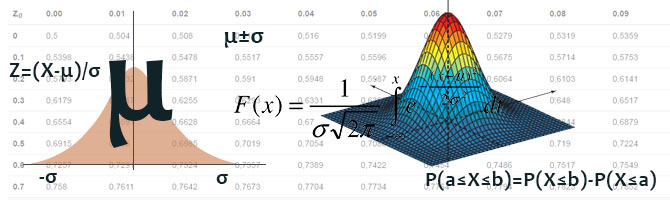
P(a ≤ X≤b) = P(X≤ b) - P(X≤ a)

The probability distribution is a function in which for each event X is assigned the probability p that the event will occur

Gaussian distribution

The normal distribution got its name absolutely fairly: according to statistics, most events occur precisely with the probability of a normal distribution, but what does this mean? It means, for example, what if you see on the bread package the designation "Weight: 400 ± 16g" - the weight of the loaf it has a normal distribution with an average value of 400g and a standard deviation of 16g.

Normal distribution table

The normal distribution table is the tabulated values of the normal distribution function.

To find the probability of an event Z₀, you can use the normal distribution table below. At the intersection of rows (n) and columns (m) is the probability value n+m.

Z₀	0.00	0.01	0.02	0.03	0.04	0.05	0.06	0.07	0.08	0.09
0	0.500	0.504	0.508	0.512	0.516	0.520	0.524	0.528	0.532	0.536
0.1	0.540	0.544	0.548	0.552	0.556	0.560	0.564	0.568	0.571	0.575
0.2	0.579	0.583	0.587	0.591	0.595	0.599	0.603	0.606	0.610	0.614
0.3	0.618	0.622	0.625	0.629	0.633	0.637	0.641	0.644	0.648	0.652
0.4	0.655	0.659	0.663	0.666	0.670	0.674	0.677	0.681	0.684	0.688
0.5	0.692	0.695	0.699	0.702	0.705	0.709	0.712	0.716	0.719	0.722
0.6	0.726	0.729	0.732	0.736	0.739	0.742	0.745	0.749	0.752	0.755
0.7	0.758	0.761	0.764	0.767	0.770	0.773	0.776	0.779	0.782	0.785
0.8	0.788	0.791	0.794	0.797	0.799	0.802	0.805	0.808	0.811	0.813
0.9	0.816	0.819	0.821	0.824	0.826	0.829	0.832	0.834	0.837	0.839
1	0.841	0.844	0.846	0.849	0.851	0.853	0.855	0.858	0.860	0.862
1.1	0.864	0.867	0.869	0.871	0.873	0.875	0.877	0.879	0.881	0.883
1.2	0.885	0.887	0.889	0.891	0.892	0.894	0.896	0.898	0.900	0.901
1.3	0.903	0.905	0.907	0.908	0.910	0.911	0.913	0.915	0.916	0.918
1.4	0.919	0.921	0.922	0.924	0.925	0.926	0.928	0.929	0.931	0.932
1.5	0.933	0.934	0.936	0.937	0.938	0.939	0.941	0.942	0.943	0.944
1.6	0.945	0.946	0.947	0.948	0.950	0.951	0.952	0.953	0.954	0.955
1.7	0.955	0.956	0.957	0.958	0.959	0.960	0.961	0.962	0.963	0.963
1.8	0.964	0.965	0.966	0.966	0.967	0.968	0.969	0.969	0.970	0.971
1.9	0.971	0.972	0.973	0.973	0.974	0.974	0.975	0.976	0.976	0.977
2	0.977	0.978	0.978	0.979	0.979	0.980	0.980	0.981	0.981	0.982
2.1	0.982	0.983	0.983	0.983	0.984	0.984	0.985	0.985	0.985	0.986
2.2	0.986	0.986	0.987	0.987	0.988	0.988	0.988	0.988	0.989	0.989
2.3	0.989	0.990	0.990	0.990	0.990	0.991	0.991	0.991	0.991	0.992
2.4	0.992	0.992	0.992	0.993	0.993	0.993	0.993	0.993	0.993	0.994
2.5	0.994	0.994	0.994	0.994	0.995	0.995	0.995	0.995	0.995	0.995
2.6	0.995	0.996	0.996	0.996	0.996	0.996	0.996	0.996	0.996	0.996
2.7	0.997	0.997	0.997	0.997	0.997	0.997	0.997	0.997	0.997	0.997
2.8	0.997	0.998	0.998	0.998	0.998	0.998	0.998	0.998	0.998	0.998
2.9	0.998	0.998	0.998	0.998	0.998	0.998	0.999	0.999	0.999	0.999
3	0.999	0.999	0.999	0.999	0.999	0.999	0.999	0.999	0.999	0.999
3.1	0.999	0.999	0.999	0.999	0.999	0.999	0.999	0.999	0.999	0.999
3.2	0.999	0.999	0.999	0.999	0.999	0.999	0.999	0.999	0.999	1.000
Table 1. Normal distribution table. Frequently used values are highlighted in red when selecting the critical area

Normal distribution - mean 0 and deviation 1?

Not only. The normal distribution graph is constructed for the mean value of zero and the standard deviation of one, i.e. 0±1. But if your mean and deviation differ from zero and one, then the following formula is at your service:

Z = (X - μ) / σ

Where μ and σ are the mean and standard deviation for your distribution, respectively, and X is the value for which you want find out the probability. Returning to the example with a loaf of bread - in order to find out what the probability is that the loaf will weigh less than 396 grams - it is necessary to substitute the values X=396, μ = 400, σ = 16 into the formula:

Z = (396 - 400) / 16 = -0.25

Next, the table needs to find the value for Z. For both Z = -0.25 and Z = 0.25, it will be 0.5987 (the normal distribution is symmetric, so the probability value is determined for the absolute value of Z: the graph is symmetric about the Y axis, so the probability value does not depend on the sign of X)

Onytabel>

Onytand Z

P ( -0.34 ≤ Z ≤ 0.34)

Z_a

Z_b

&muinput type="text" class="form-control" id="normal_distr_m" value="0"/>

σ (fromklheenie)

Properties of the distribution function

Symmetrical with respect to the center (the average value is the mathematical expectation)
The mode and median are equal to the mathematical expectation μ

Distribution function

The distribution function is designed to determine what is the probability that the value of X is less than or equal to some number of x.

Using the example of the loaf from the first paragraph: if we want to find out what is the probability that the loaf will weigh less than 410 grams, then using by the reduction formula, we get Z=0.63 and the value of P(X<0.63) = 0.7357, i.e. the probability that the loaf will weigh 410 grams or less - 73.57%

The average value of the normal distribution (μ)

The mathematical expectation (average value) for a standard normal distribution is zero: μ = 0

Normal distribution in excel

To get the value of the normal distribution in excel, there is a formula "NORMS.RASP" (in old versions of the NORM), to which is passed the value of the event X, for example, what is the probability of getting into the interval [-0.5;0.5]?

=NORMAL(0,5;0;1;1) = 0,35< br/> =NORM.RASP(0,5;0;1;1) = 0,35 < br/>

The syntax of the command is as follows: NORMAL (event X, mean, deviation, integral). So, you can find the value normal distribution without reduction of values:

=NORM.RASP(396;400;16;1) = 0.4
To find the value of Z, if there is a probability, for example, for 95%, you can use the formula "NORMOBR":

=NORMOBR(0.95;0;1) = 1.64

Tests

Normal distribution

Download article in PDF format.

Author: Zakhar Telyatnikov
Last edit time: 23.10.2023

24.10.2016

Do you find this article curious? /

Seen: 40 669

Read the following
Poisson distribution